《電力》〈送電〉[R06下:問12]三相3線式送電線の電力損失率に関する計算問題

【問題】

【難易度】★★☆☆☆（やや易しい）

三相\( \ 3 \ \)線式交流送電線があり，電線\( \ 1 \ \)線当たりの抵抗が\( \ R \ \mathrm {[\Omega ]} \ \)，受電端の線間電圧が\( \ V_{\mathrm {r}} \ \mathrm {[V]} \ \)である。今，受電端から力率\( \ \cos \theta \ \)の負荷に三相電力\( \ P \ \mathrm {[W]} \ \)を供給しているものとする。

この送電線での\( \ 3 \ \)線の電力損失を\( \ P_{\mathrm {L}} \ \)とすると，電力損失率\( \ \displaystyle \frac {P_{\mathrm {L}}}{P} \ \)を表す式として，正しいものを次の(1)～(5)のうちから一つ選べ。

ただし，線路のインダクタンス，静電容量及びコンダクタンスは無視できるものとする。

　(1)　\( \ \displaystyle \frac {RP}{3\left( V_{\mathrm {r}}\cos \theta \right) ^{2}} \ \)　　(2)　\( \ \displaystyle \frac {RP}{\left( V_{\mathrm {r}}\cos \theta \right) ^{2}} \ \)　　(3)　\( \ \displaystyle \frac {RP^{2}}{\left( V_{\mathrm {r}}\cos \theta \right) ^{2}} \ \)
　(4)　\( \ \displaystyle \frac {3RP}{\left( V_{\mathrm {r}}\cos \theta \right) ^{2}} \ \)　　(5)　\( \ \displaystyle \frac {3RP^{2}}{\left( V_{\mathrm {r}}\cos \theta \right) ^{2}} \ \)

【ワンポイント解説】

三相\( \ 3 \ \)線式交流送電線の電力損失率を求める問題です。
重要公式である電力の公式\( \ P =\sqrt {3}V_{\mathrm {r}}I\cos \theta \ \)と電力損失\( \ P_{\mathrm {L}} =3rI^{2} \ \)を組み合わせて導出します。
計算慣れのような問題なので，ぜひ本問で理解するようにして下さい。
本問は平成19年問10からの再出題となります。

1.送電線の送電電力と電力損失
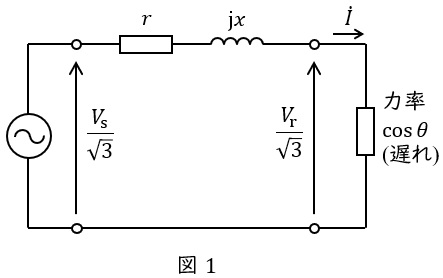
送電端の電圧が\( \ V_{\mathrm {s}} \ \mathrm {[V]} \ \)，受電端の電圧が\( \ V_{\mathrm {r}} \ \mathrm {[V]} \ \)，送電線の\( \ 1 \ \)線あたりの抵抗が\( \ r \ \mathrm {[\Omega ]} \ \)，リアクタンスが\( \ x \ \mathrm {[\Omega ]} \ \)，送電線に流れる電流が\( \ I \ \mathrm {[A]} \ \)，負荷の力率が\( \ \cos \theta \ \)であるとすると，送電線の一相分等価回路は図1のように描くことができます。

このとき，負荷電力\( \ P \ \mathrm {[W]} \ \)は，
\[
\begin{eqnarray}
P &=&\sqrt {3}V_{\mathrm {r}}I\cos \theta \\[ 5pt ] \end{eqnarray}
\] となり，上式より，
\[
\begin{eqnarray}
I &=&\frac {P}{\sqrt {3}V_{\mathrm {r}}\cos \theta } \\[ 5pt ] \end{eqnarray}
\] であるため，送電線での電力損失\( \ P_{\mathrm {L}} \ \mathrm {[W]} \ \)は，
\[
\begin{eqnarray}
P_{\mathrm {L}} &=&3rI^{2} \\[ 5pt ] &=&3r\left( \frac {P}{\sqrt {3}V_{\mathrm {r}}\cos \theta }\right) ^{2} \\[ 5pt ] &=&\frac {P^{2}r}{V_{\mathrm {r}}^{2}\cos ^{2}\theta } \\[ 5pt ] \end{eqnarray}
\] となります。

【解答】

解答：(2)
送電線での電力損失\( \ P_{\mathrm {L}} \ \mathrm {[W]} \ \)は，ワンポイント解説「1.送電線の送電電力と電力損失」の通り，
\[
\begin{eqnarray}
P_{\mathrm {L}} &=&\frac {RP^{2}}{V_{\mathrm {r}}^{2}\cos ^{2}\theta } \\[ 5pt ] \end{eqnarray}
\] であるから，電力損失率\( \ \displaystyle \frac {P_{\mathrm {L}}}{P} \ \)は，
\[
\begin{eqnarray}
\frac {P_{\mathrm {L}}}{P} &=&\frac {\displaystyle \frac {RP^{2}}{V_{\mathrm {r}}^{2}\cos ^{2}\theta }}{P} \\[ 5pt ] &=&\frac {RP}{\left( V_{\mathrm {r}}\cos \theta \right) ^{2}} \\[ 5pt ] \end{eqnarray}
\] と求められる。