《理論》〈電気回路〉[H22:問15]Δ結線した三相平衡回路の消費電力に関する計算問題

【問題】

【難易度】★★★☆☆（普通）

図の平衡三相回路について，次の(a)及び(b)に答えよ。

(a)　端子$\ \mathrm {a} \ $，$\ \mathrm {c} \ $に$\ 100 \ \mathrm {[V]} \ $の単相交流電源を接続したところ、回路の消費電力は$\ 200 \ \mathrm {[W]} \ $であった。抵抗$\ R \ \mathrm {[\Omega ]} \ $の値として，正しいのは次のうちどれか。

　(1)　$\ 0.30 \ $　　(2)　$\ 30 \ $　　(3)　$\ 33 \ $　　(4)　$\ 50 \ $　　(5)　$\ 83 \ $

(b)　端子$\ \mathrm {a} \ $，$\ \mathrm {b} \ $，$\ \mathrm {c} \ $に線間電圧$\ 200 \ \mathrm {[V]} \ $の対称三相交流電源を接続したときの全消費電力$\ \mathrm {[kW]} \ $の値として，正しいのは次のうちどれか。

　(1)　$\ 0.48 \ $　　(2)　$\ 0.80 \ $　　(3)　$\ 1.2 \ $　　(4)　$\ 1.6 \ $　　(5)　$\ 4.0 \ $

【ワンポイント解説】

三相平衡回路における抵抗の消費電力に関する問題です。
$\ 3 \ $種では圧倒的に三相平衡回路が出題されやすいので，三相平衡回路の$\ \Delta – \mathrm {Y} \ $変換は必ずマスターしておきましょう。

1.合成抵抗
抵抗$\ R_{1} \ \mathrm {[\Omega ]} \ $と$\ R_{2} \ \mathrm {[\Omega ]} \ $が与えられている時，それぞれの合成抵抗$\ R \ \mathrm {[\Omega ]} \ $は以下の式で与えられます。

①直列
直列合成抵抗$\ R \ \mathrm {[\Omega ]} \ $は，
$$\begin{eqnarray} R&=&R_{1}+R_{2} \\[ 5pt ] \end{eqnarray}$$ となります。

②並列
並列合成抵抗$\ R \ \mathrm {[\Omega ]} \ $は，
$$\begin{eqnarray} \frac {1}{R}&=&\frac {1}{R_{1}}+\frac {1}{R_{2}} \\[ 5pt ] \end{eqnarray}$$ となり，整理すると，
$$\begin{eqnarray} R&=&\frac {R_{1}R_{2}}{R_{1}+R_{2}} \\[ 5pt ] \end{eqnarray}$$ となります。

2.$\ \Delta -\mathrm {Y} \ $変換と$\ \mathrm {Y}-\Delta \ $変換
①$\ \Delta -\mathrm {Y} \ $変換
図1において，
$$\begin{eqnarray} {\dot Z}_{\mathrm {a}}&=&\frac {{\dot Z}_{\mathrm {ab}}{\dot Z}_{\mathrm {ca}}}{{\dot Z}_{\mathrm {ab}}+{\dot Z}_{\mathrm {bc}}+{\dot Z}_{\mathrm {ca}}} \\[ 5pt ] {\dot Z}_{\mathrm {b}}&=&\frac {{\dot Z}_{\mathrm {bc}}{\dot Z}_{\mathrm {ab}}}{{\dot Z}_{\mathrm {ab}}+{\dot Z}_{\mathrm {bc}}+{\dot Z}_{\mathrm {ca}}} \\[ 5pt ] {\dot Z}_{\mathrm {c}}&=&\frac {{\dot Z}_{\mathrm {ca}}{\dot Z}_{\mathrm {bc}}}{{\dot Z}_{\mathrm {ab}}+{\dot Z}_{\mathrm {bc}}+{\dot Z}_{\mathrm {ca}}} \\[ 5pt ] \end{eqnarray}$$ ②$\ \mathrm {Y}-\Delta \ $変換
図1において，
$$\begin{eqnarray} {\dot Z}_{\mathrm {ab}}&=&\frac {{\dot Z}_{\mathrm {a}}{\dot Z}_{\mathrm {b}}+{\dot Z}_{\mathrm {b}}{\dot Z}_{\mathrm {c}}+{\dot Z}_{\mathrm {c}}{\dot Z}_{\mathrm {a}}}{{\dot Z}_{\mathrm {c}}} \\[ 5pt ] {\dot Z}_{\mathrm {bc}}&=&\frac {{\dot Z}_{\mathrm {a}}{\dot Z}_{\mathrm {b}}+{\dot Z}_{\mathrm {b}}{\dot Z}_{\mathrm {c}}+{\dot Z}_{\mathrm {c}}{\dot Z}_{\mathrm {a}}}{{\dot Z}_{\mathrm {a}}} \\[ 5pt ] {\dot Z}_{\mathrm {ca}}&=&\frac {{\dot Z}_{\mathrm {a}}{\dot Z}_{\mathrm {b}}+{\dot Z}_{\mathrm {b}}{\dot Z}_{\mathrm {c}}+{\dot Z}_{\mathrm {c}}{\dot Z}_{\mathrm {a}}}{{\dot Z}_{\mathrm {b}}} \\[ 5pt ] \end{eqnarray}$$ 平衡三相回路においては，
$${\dot Z}_{\mathrm {ab}}={\dot Z}_{\mathrm {bc}}={\dot Z}_{\mathrm {ca}}=3{\dot Z}_{\mathrm {a}}=3{\dot Z}_{\mathrm {b}}=3{\dot Z}_{\mathrm {c}}$$ となります。

3.抵抗での消費電力
ある抵抗$\ R \ \mathrm {[\Omega ]} \ $に電圧$\ V \ \mathrm {[V]} \ $をかけたとき抵抗に電流$\ I \ \mathrm {[A]} \ $が流れたとすると，$\ R \ \mathrm {[\Omega ]} \ $での消費電力$\ P \ \mathrm {[W]} \ $は，
$$\begin{eqnarray} P &=&VI \\[ 5pt ] \end{eqnarray}$$ となります。オームの法則$\ V=RI \ $より上式は，
$$\begin{eqnarray} P &=&RI^{2} \\[ 5pt ] &=&\frac {V^{2}}{R} \\[ 5pt ] \end{eqnarray}$$ と変形できます。

【解答】

(a)解答：(2)
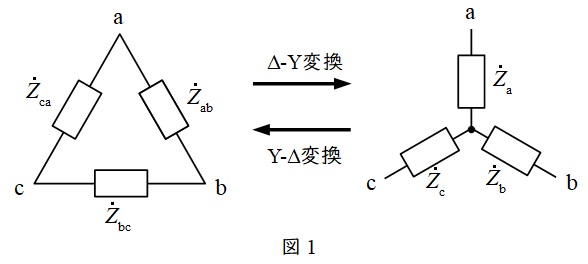
ワンポイント解説「2.$\ \Delta -\mathrm {Y} \ $変換と$\ \mathrm {Y}-\Delta \ $変換」の通り，問題図を$\ \Delta -\mathrm {Y} \ $変換すると図2のようになる。
端子$\ \mathrm {a} \ $，$\ \mathrm {c} \ $に$\ V=100 \ \mathrm {[V]} \ $の電源を繋ぐと，電流が流れるのは図3に示した箇所であるため，このときの合成抵抗$\ R_{0} \ \mathrm {[\Omega ]} \ $は，ワンポイント解説「1.合成抵抗」の通り，
$$\begin{eqnarray} R_{\mathrm {0}}&=&\frac {R}{2}+\frac {R}{3}+\frac {R}{3}+\frac {R}{2} \\[ 5pt ] &=&\frac {5R}{3} \\[ 5pt ] \end{eqnarray}$$ なり，このときに回路で消費される電力が$\ P=200 \ \mathrm {[W]} \ $であるから，ワンポイント解説「3.抵抗での消費電力」より，
$$\begin{eqnarray} P &=&\frac {V^{2}}{R_{\mathrm {0}}} \\[ 5pt ] R_{\mathrm {0}} &=&\frac {V^{2}}{P} \\[ 5pt ] \frac {5R}{3}&=&\frac {V^{2}}{P} \\[ 5pt ] R&=&\frac {3V^{2}}{5P} \\[ 5pt ] &=&\frac {3\times 100^{2}}{5\times 200} \\[ 5pt ] &=&30 \ \mathrm {[\Omega ]} \\[ 5pt ] \end{eqnarray}$$ と求められる。

(b)解答：(4)
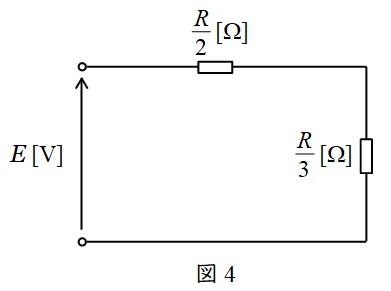
題意に沿って一相分等価回路を描くと図4のようになる。
対称三相交流電源の相電圧は$\ \displaystyle E=\frac {200}{\sqrt {3}} \ \mathrm {[V]} \ $であり，一相あたりの抵抗$\ R_{1} \ \mathrm {[\Omega ]} \ $は，ワンポイント解説「1.合成抵抗」の通り，
$$\begin{eqnarray} R_{\mathrm {1}}&=&\frac {R}{2}+\frac {R}{3} \\[ 5pt ] &=&\frac {5R}{6} \\[ 5pt ] &=&\frac {5\times 30}{6} \\[ 5pt ] &=&25 \ \mathrm {[\Omega ]} \\[ 5pt ] \end{eqnarray}$$ であるため，全消費電力$\ P_{3} \ \mathrm {[kW]} \ $は，
$$\begin{eqnarray} P_{3}&=&3\cdot \frac {E^{2}}{R_{\mathrm {1}}} \\[ 5pt ] &=&3\times \frac {\displaystyle \left( \frac {200}{\sqrt {3}}\right) ^{2}}{25} \\[ 5pt ] &=&1 \ 600 \ \mathrm {[W]}　→　1.6 \ \mathrm {[kW]} \\[ 5pt ] \end{eqnarray}$$ と求められる。