《理論》〈電気回路〉[H24:問16]三相回路の相電流及び線電流に関する計算問題

Contents

1 【問題】
2 【ワンポイント解説】
3 【解答】

【問題】

【難易度】★★★★☆（やや難しい）

図のように，相電圧\( \ 200 \ \mathrm {[V]} \ \)の対称三相交流電源に，複素インピーダンス\( \ \dot Z =5\sqrt {3}+\mathrm {j}5 \ \mathrm {[\Omega ]} \ \)の負荷が\( \ \mathrm {Y} \ \)結線された平衡三相負荷を接続した回路がある。
次の(a)及び(b)の問に答えよ。

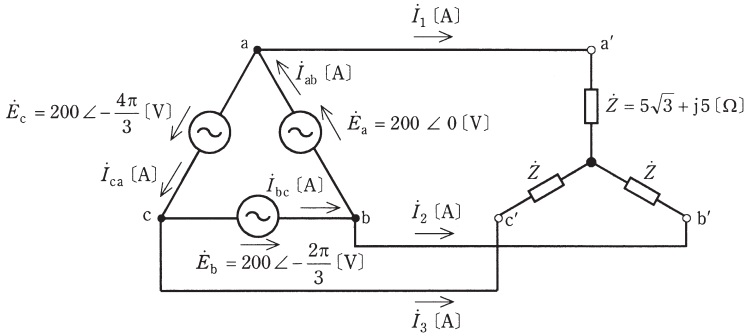
(a)　電流\( \ {\dot I}_{1} \ \mathrm {[A]} \ \)の値として，最も近いものを次の(1)～(5)のうちから一つ選べ。

　(1)　\( \ 20.00 \ ∠-\displaystyle \frac {\pi }{3} \ \)　　(2)　\( \ 20.00 \ ∠-\displaystyle \frac {\pi }{6} \ \)　　(3)　\( \ 16.51 \ ∠-\displaystyle \frac {\pi }{6} \ \)

　(4)　\( \ 11.55 \ ∠-\displaystyle \frac {\pi }{3} \ \)　　(5)　\( \ 11.55 \ ∠-\displaystyle \frac {\pi }{6} \ \)

(b)　電流\( \ {\dot I}_{\mathrm {ab}} \ \mathrm {[A]} \ \)の値として，最も近いものを次の(1)～(5)のうちから一つ選べ。

　(1)　\( \ 20.00 \ ∠-\displaystyle \frac {\pi }{6} \ \)　　(2)　\( \ 11.55 \ ∠-\displaystyle \frac {\pi }{3} \ \)　　(3)　\( \ 11.55 \ ∠-\displaystyle \frac {\pi }{6} \ \)

　(4)　\( \ 6.67 \ ∠-\displaystyle \frac {\pi }{3} \ \ \ \)　　(5)　\( \ 6.67 \ ∠-\displaystyle \frac {\pi }{6} \ \)　　　　

【ワンポイント解説】

\( \ \mathrm {\Delta – Y } \ \)変換及び\( \ \mathrm {Y – \Delta } \ \)変換，相電圧と線間電圧の関係，線電流と相電流の関係等すべてを理解していることが求められる問題です。演習としてはとても良い問題と思います。

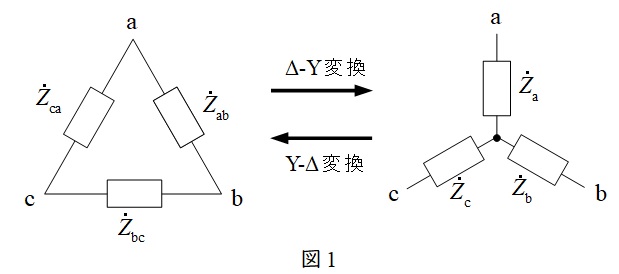
1.\( \ \Delta -\mathrm {Y} \ \)変換と\( \ \mathrm {Y}-\Delta \ \)変換
①\( \ \Delta -\mathrm {Y} \ \)変換
図1において，
\[
\begin{eqnarray}
{\dot Z}_{\mathrm {a}}&=&\frac {{\dot Z}_{\mathrm {ab}}{\dot Z}_{\mathrm {ca}}}{{\dot Z}_{\mathrm {ab}}+{\dot Z}_{\mathrm {bc}}+{\dot Z}_{\mathrm {ca}}} \\[ 5pt ] {\dot Z}_{\mathrm {b}}&=&\frac {{\dot Z}_{\mathrm {bc}}{\dot Z}_{\mathrm {ab}}}{{\dot Z}_{\mathrm {ab}}+{\dot Z}_{\mathrm {bc}}+{\dot Z}_{\mathrm {ca}}} \\[ 5pt ] {\dot Z}_{\mathrm {c}}&=&\frac {{\dot Z}_{\mathrm {ca}}{\dot Z}_{\mathrm {bc}}}{{\dot Z}_{\mathrm {ab}}+{\dot Z}_{\mathrm {bc}}+{\dot Z}_{\mathrm {ca}}} \\[ 5pt ] \end{eqnarray}
\] ②\( \ \mathrm {Y}-\Delta \ \)変換
図1において，
\[
\begin{eqnarray}
{\dot Z}_{\mathrm {ab}}&=&\frac {{\dot Z}_{\mathrm {a}}{\dot Z}_{\mathrm {b}}+{\dot Z}_{\mathrm {b}}{\dot Z}_{\mathrm {c}}+{\dot Z}_{\mathrm {c}}{\dot Z}_{\mathrm {a}}}{{\dot Z}_{\mathrm {c}}} \\[ 5pt ] {\dot Z}_{\mathrm {bc}}&=&\frac {{\dot Z}_{\mathrm {a}}{\dot Z}_{\mathrm {b}}+{\dot Z}_{\mathrm {b}}{\dot Z}_{\mathrm {c}}+{\dot Z}_{\mathrm {c}}{\dot Z}_{\mathrm {a}}}{{\dot Z}_{\mathrm {a}}} \\[ 5pt ] {\dot Z}_{\mathrm {ca}}&=&\frac {{\dot Z}_{\mathrm {a}}{\dot Z}_{\mathrm {b}}+{\dot Z}_{\mathrm {b}}{\dot Z}_{\mathrm {c}}+{\dot Z}_{\mathrm {c}}{\dot Z}_{\mathrm {a}}}{{\dot Z}_{\mathrm {b}}} \\[ 5pt ] \end{eqnarray}
\] 平衡三相回路においては，
\[
{\dot Z}_{\mathrm {ab}}={\dot Z}_{\mathrm {bc}}={\dot Z}_{\mathrm {ca}}=3{\dot Z}_{\mathrm {a}}=3{\dot Z}_{\mathrm {b}}=3{\dot Z}_{\mathrm {c}}
\] となります。

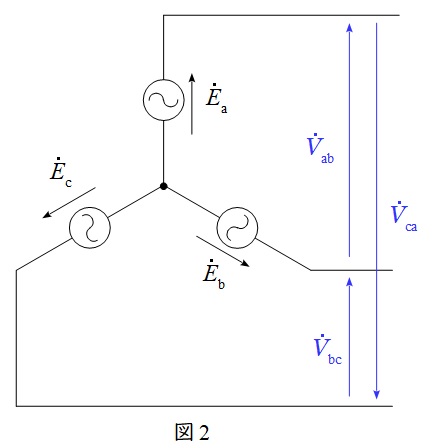
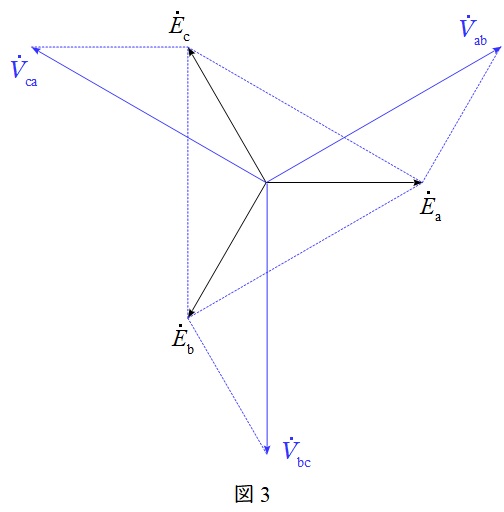
2.相電圧と線間電圧の関係
図2のような三相対称電源がある時，線間電圧との関係は図3のベクトル図のようになり，線間電圧の大きさ\( \ V \ \)は相電圧の大きさ\( \ E \ \)と比較すると，
\[
\begin{eqnarray}
V &=&\sqrt {3}E \\[ 5pt ] \end{eqnarray}
\] かつ\( \ \displaystyle \frac {\pi }{6} \ \)（30°)進みであることが分かります。

【解答】

(a)解答：(4)
ワンポイント解説「2.相電圧と線間電圧の関係」より，\( \ \mathrm {a} \ \)点での相電圧\( \ E_{\mathrm {a}}^{\prime } \ \)は，
\[
\begin{eqnarray}
E_{\mathrm {a}}^{\prime } &=&\frac {200}{\sqrt {3}}∠-\frac {\pi }{6} \\[ 5pt ] \end{eqnarray}
\] である。また，インピーダンス\( \ \dot Z \ \)の大きさ\( \ Z \ \)は，
\[
\begin{eqnarray}
Z &=&\sqrt {\left( 5\sqrt {3}\right) ^{2}+5^{2}} \\[ 5pt ] &=&10 \ \mathrm {[\Omega ]} \\[ 5pt ] \end{eqnarray}
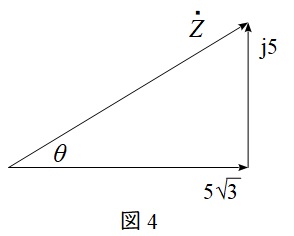
\] となる。よって，\( \ \dot Z \ \)をベクトル図で表すと，図4のようになり，力率角は\( \ \displaystyle \frac {\pi }{6} \ \)であることが分かる。

したがって，電流\( \ {\dot I}_{1} \ \)は，
\[
\begin{eqnarray}
{\dot I}_{1} &=&\frac {{\dot E}_{\mathrm {a}}^{\prime }}{\dot Z} \\[ 5pt ] &=&\left| \frac {{\dot E}_{\mathrm {a}}^{\prime }}{\dot Z}\right| ∠ \left( -\frac {\pi }{6}-\frac {\pi }{6}\right) \\[ 5pt ] &=&\left| \frac {\displaystyle \frac {200}{\sqrt {3}}}{10}\right| ∠ \left( -\frac {\pi }{6}-\frac {\pi }{6}\right) \\[ 5pt ] &≒&11.55∠ -\frac {\pi }{3} \ \mathrm {[A]} \\[ 5pt ] \end{eqnarray}
\] と求められる。

(b)解答：(5)
ワンポイント解説「1.\( \ \Delta -\mathrm {Y} \ \)変換と\( \ \mathrm {Y}-\Delta \ \)変換」の通り，負荷側を\( \ \mathrm {Y}-\Delta \ \)変換すると，
\[
\begin{eqnarray}
Z_{\mathrm {ab}} &=&3Z \\[ 5pt ] &=&3\times 10 \\[ 5pt ] &=&30 \ \mathrm {[\Omega ]} \\[ 5pt ] \end{eqnarray}
\] であるから，\( \ {\dot I}_{\mathrm {ab}} \ \)は，
\[
\begin{eqnarray}
{\dot I}_{\mathrm {ab}} &=&\frac {{\dot E}_{\mathrm {a}}}{{\dot Z}_{\mathrm {ab}}} \\[ 5pt ] &=&\left| \frac {{\dot E}_{\mathrm {a}}}{{\dot Z}_{\mathrm {ab}}}\right| ∠ \left( 0-\frac {\pi }{6}\right) \\[ 5pt ] &=&\left| \frac {200}{30}\right| ∠ \left( 0-\frac {\pi }{6}\right) \\[ 5pt ] &≒&6.67∠ -\frac {\pi }{6} \ \mathrm {[A]} \\[ 5pt ] \end{eqnarray}
\] と求められる。